The future value of any annuity equals the sum of the future values for all of the annuity payments when they are moved to the end of the last payment interval. For example, assume you will make [latex]\$1,000[/latex] contributions at the end of every year for the next three years to an investment earning [latex]10\%[/latex] compounded annually. A lottery winner could use an annuity table to determine whether it makes more financial sense to take their lottery winnings as a lump-sum payment today, or as a series of payments over many years. More commonly, annuities are a type of investment used to provide individuals with a steady income in retirement. As with future value calculations, calculating present values by manually moving each payment to its present value is extremely time consuming when there are more than a few payments. Similarly, annuity formulas allow you to move all payments simultaneously in a single calculation.
What Is the Difference Between an Ordinary Annuity and an Annuity Due?

It takes into account the amount of money that has been placed in the annuity and how long it’s been sitting there, so as to decide the amount of money that should be paid out to an annuity buyer or annuitant. Using the previous inputs, fill in the interest rate of 0.05, the time period of 3 (years), and payments of -100. If the formula doesn’t automatically calculate, go to the right-hand side of the worksheet at the top and click on Calculate to get the answer of $272.32.
How We Make Money
This problem involves an annuity (the yearly net cash flows of $10,000) and a single amount (the $250,000 to be received once at the end of the twentieth year). For example, suppose that you are considering purchasing an apartment. After much deliberation, you determine that you will receive net yearly cash flows of $10,000 from rental revenue, less rental expenses from the apartment. To demonstrate how to calculate the present value of an annuity, assume that you are offered an investment that pays $2,000 a year at the end of each of the next 10 years. Suppose you want to determine the value today of receiving $1.00 at the end of each of the next 4 years. To solve this, we can construct a table that determines the present values of each of the receipts.
How to calculate the present value of an annuity due
- If you’d like to learn more about the Net Present Value (and other investment appraisal or capital budgeting techniques), do check out our course on Investment Appraisal Mastery.
- In our illustrative example, we’ll calculate an annuity’s present value (PV) under two different scenarios.
- In this specific case, the Present Value of an Annuity Factor is the number we multiply the cash flow by, in order to calculate the Present Value of an Annuity.
- Besides, you can find the annuity formulas and get some insight into their mathematical background.
- The first involves a present value annuity calculation using Formula 11.4.
It’s true that $100,000 in your pocket today is worth more than 10 payments of $10,000 over 10 years. However, this assumes you’ll invest the $100,000 and let it grow for 10 years. A few factors that affect your annuity’s value include the interest rate, payment amount, payment period, and fees. Keep in mind that the formulas in this article assume a fixed rate of return. For indexed and variable annuities, the interest rate would be an estimate based on expectations in the market. Now as that you know all the financial terms appearing in this calculator, let’s do a quick example of how the annuity formulas can be applied.
Retirement
For example, payments scheduled to arrive in the next five years are worth more than payments scheduled 25 years in the future. Earlier cash flows can be reinvested earlier and for a longer duration, so these cash flows carry the highest value (and vice versa for cash flows received later). The reason the values are higher is that payments made at the beginning of the period have more time to earn interest. For example, if the $1,000 was invested on January 1 rather than January 31, it would have an additional month to grow. So, for example, if you plan to invest a certain amount each month or year, FV will tell you how much you will accumulate as of a future date. If you are making regular payments on a loan, the FV is useful in determining the total cost of the loan.
For example, you can use it either for regular deposits or withdrawals, for multiple frequencies, or you can compare ordinary annuity vs. annuity due. If you want to compute today’s present value of a single lump sum payment (instead of series of payments) in the future than try our present value calculator here. In the rare circumstance where the final payment is exactly equal to all other annuity payments, you can arrive at the balance owing through a present value annuity calculation. In this instance, since you are starting at the end of the loan, the future value is always zero, so to bring all payments back to the focal date you only need Formula 11.4. Observe that only two of the three payments need to be present valued to your focal date since the first payment is already on the focal date.
You’ll see a dialogue box open with spaces for you to fill in the information for your PV calculation. For this reason, we created the calculator for instructional purposes only. Still, if you experience a relevant drawback or encounter any inaccuracy, we are always pleased to receive useful feedback and advice. Second, you’ll need to find out how much you’ll need to invest today to make that happen.
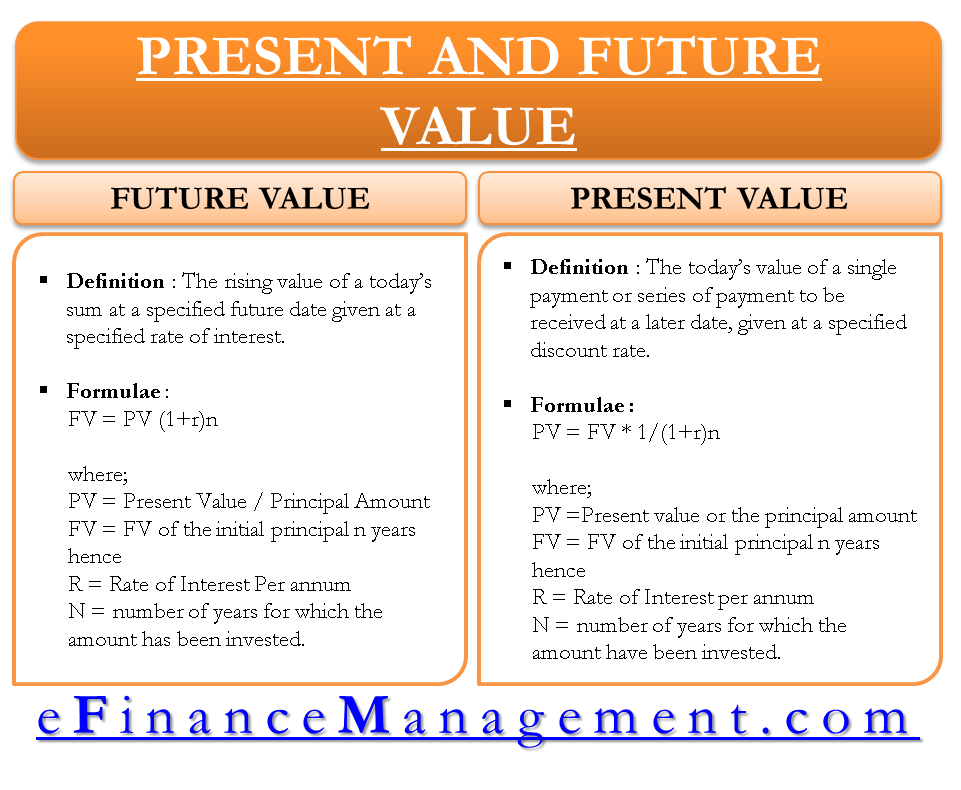
The present value (PV) of an annuity is the current value of future payments from an annuity, given a specified rate of return or discount rate. It is calculated using a formula that takes into account the time value of money and the discount rate, which is an assumed rate of return or interest rate over the same duration as the payments. The present value of an annuity can be used to determine whether it is more beneficial to receive a lump-sum payment or an annuity spread out over a number of years.
The total investment for an annuity due is higher at $2,735.54 because the first payment is withdrawn immediately, so a smaller principal earns less interest than does the ordinary annuity. This section develops present value formulas for both ordinary annuities and annuities due. Like future value calculations, these formulas accommodate both simple and general annuities as needed. From investments, we will then extend annuity calculations to loans as well.
The formulas for ordinary annuities and annuities due are presented together. The future value of an ordinary annuity tells you how much your account would be worth after an accumulation phase when you make contributions. In this case, you’re investing money what are the tax benefits of homeownership 2020 to receive the benefit of compounding interest. Each year after the first year, you get an interest payment from the annuity. The interest that is generated on annuities is tax-deferred, so there is no tax due on the growth until the time of withdrawal.
